Vector product

A vector product, also known as cross product, is an antisymmetric product A × B = −B × A of two vectors A and B in 3-dimensional Euclidean space $\scriptstyle \mathbb {R} ^{3}$. The vector product is again a 3-dimensional vector. The vector product is widely used in many areas of mathematics, mechanics, electromagnetism, gravitational fields, etc.

Definition

Given two vectors, A and B in $\scriptstyle \mathbb {R} ^{3}$, the vector product is a vector with length AB sin θ_AB, where A is the length of A, B is the length of B, and θ_AB is the smaller (non-reentrant) angle between A and B. The direction of the vector product is perpendicular (or normal to) the plane containing the vectors A and B and follows the right-hand rule (see below),

$\mathbf {A} \times \mathbf {B} =\mathbf {a} _{\textrm {N}}\,|\mathbf {A} |\,|\mathbf {B} |\,\sin \theta _{AB},$

where a_N is a unit vector normal to the plane spanned by A and B in the right-hand rule direction.

We recall that the length of a vector is the square root of the dot product of a vector with itself, A ≡ |A| = (A ⋅ A )^1/2 and similarly for the length of B. A unit vector has by definition length one.

From the antisymmetry A × B = −B × A follows that the cross (vector) product of any vector with itself (or another parallel or antiparallel vector) is zero because A × A = − A × A and the only quantity equal to minus itself is the zero. Alternatively, one may derive this from the fact that sin(0) = 0 (parallel vectors) and sin(180) = 0 (antiparallel vectors).

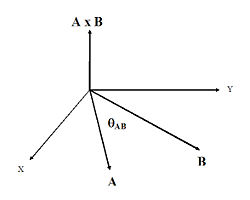
The right hand rule

Fig. 1. Diagram illustrating the direction of A×B. The plane containing A and B is the x-y plane, A×B is along the z-axis.

The diagram in Fig. 1 illustrates the direction of A × B, which follows the right-hand rule. If one points the fingers of the right hand towards the head of vector A (with the wrist at the origin), then curls them towards the direction of B, the extended thumb will point in the direction of A × B.

Another formulation of the cross product

Rather than from the angle and perpendicular unit vector, the form of the cross product below is often used. In this definition we need to express the vectors with respect to a Cartesian (orthonormal) coordinate frame a_x, a_y and a_z of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \scriptstyle \mathbb{R}^3} . With respect to this frame we write A = (A_x, A_y, A_z) and B = (B_x, B_y, B_z). Then

A × B = (A_yB_z - A_zB_y)a_x + (A_zB_x - A_xB_z)a_y + (A_xB_y - A_yB_x)a_z,

This formula can be written more concisely upon introduction of a determinant:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbf A \times \mathbf B = \left|\begin{array}{ccc} \mathbf a_x & \mathbf a_y & \mathbf a_z \\ A_x & A_y & A_z \\ B_x & B_y & B_z \end{array} \right|, }

where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left|\cdot\right|} denotes the determinant of a matrix. This determinant must be evaluated along the first row, otherwise the equation does not make sense.

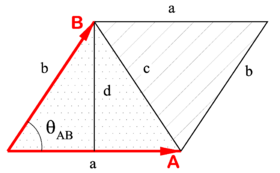
Geometric representation of the length

We repeat that the length of the cross product of vectors A and B is equal to

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\mathbf{A}\times \mathbf{B}| = |\mathbf{A}|\, |\mathbf{B}|\,\sin\theta_{AB}, }

because a_N has by definition length 1.

Fig. 2. The length of the cross product A×B is equal to area of the parallelogram with sides a and b, the sum of the areas of the dotted and dashed triangle.

Using the high school geometry rule: the area S of a triangle is its base a times its half-height d, we see in Fig. 2 that the area S of the dotted triangle is equal to:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle S = \frac{ad}{2} = \frac{|\mathbf{A}| |\mathbf{B}|\sin\theta_{AB}}{2} = \frac{|\mathbf{A}\times \mathbf{B}|}{2}, }

because, as follows from Fig. 2:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a = |\mathbf{A}| \quad\textrm{and}\quad d = b \sin\theta_{AB} = |\mathbf{B}|\sin\theta_{AB} . }

Hence |A×B| = 2S. Since the dotted triangle with sides a, b, and c is congruent to the dashed triangle, the area of the dotted triangle is equal to the area of the dashed triangle and the length 2S of the cross product is equal to the sum of the areas of the dotted and the dashed triangle. In conclusion: The area of the parallelogram spanned by the vectors A and B is equal to the length of A×B.

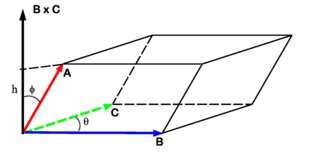
Application: the volume of a parallelepiped

The volume V of the parallelepiped shown in Fig. 3 is given by

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle V = \mathbf{A}\cdot (\mathbf{B}\times\mathbf{C}). }

Fig. 3. Parallelepiped generated by the vectors A , B , and C . Its height is h, the projection of A on B×C.

Indeed, remember that the volume of a parallelepiped is given by the area S of its base times its height h, V = Sh. Above it was shown that, if

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbf{D} \equiv \mathbf{B}\times\mathbf{C}\quad\textrm{then}\quad S = |\mathbf{D}|. }

The height h of the parallelepiped is the length of the projection of the vector A onto D. The dot product between A and D is |D| times the length h,

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbf{D}\cdot\mathbf{A}= |\mathbf{D}|\,\big(|\mathbf{A}| \cos\phi\big) = |\mathbf{D}|\, h = S h, }

so that V = (B×C)⋅A = A⋅(B×C).

It is of interest to point out that V can be given by a determinant that contains the components of A, B, and C with respect to a Cartesian coordinate system,

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{align} V &= \begin{vmatrix} A_x & B_x & C_x \\ A_y & B_y & C_y \\ A_z & B_z & C_z \\ \end{vmatrix} \\ &= A_x (B_y C_z - B_z C_y) + A_y(B_z C_x - B_x C_z) + A_z(B_x C_y - B_y C_x). \end{align} }

From the permutation properties of a determinant follows

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbf{A}\cdot (\mathbf{B}\times\mathbf{C}) = \mathbf{B}\cdot (\mathbf{C}\times\mathbf{A}) =\mathbf{C}\cdot (\mathbf{A}\times\mathbf{B}). }

Generalization

From a somewhat more abstract point of view one may define the vector product as an element of the antisymmetric subspace of the 9-dimensional tensor product space Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \scriptstyle \mathbb{R}^3 \otimes \mathbb{R}^3 } . This antisymmetric subspace is of dimension 3.

In general the antisymmetric subspace of the k-fold tensor power Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \scriptstyle \mathbb{R}^n \otimes \mathbb{R}^n\otimes \cdots\otimes\mathbb{R}^n } is of dimension Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \scriptstyle {n \choose k}} . Elements of such space are often called wedge products written as

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle A_1 \wedge A_2 \wedge A_3 \cdots \wedge A_k,\qquad A_i \in \mathbb{R}^n, \qquad i=1,\dots,k. }

The antisymmetric subspace of a two-fold tensor product space is of dimension

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle {n \choose 2} = n(n-1)/2} .

The latter number is equal to 3 only if n = 3. For instance, for n = 2 or 4, the antisymmetric subspaces are of dimension 1 and 6, respectively.

Hence, if one regards the vector product in Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \scriptstyle \mathbb{R}^3} from the point of view of antisymmetric subspaces, it is a "coincidence" that the product lies again in Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \scriptstyle \mathbb{R}^3} .

The cross product lacks the property of a proper vector that it changes sign under inversion [both factors of the cross product change sign and (−1)×(−1) = 1]. A vector that does not change sign under inversion is called an axial vector or pseudo vector. Hence a cross product is a pseudo vector. A vector that does change sign is often referred to as a polar vector in this context.